
Lotto is an in-state game that costs $1 for two plays. EZ Match is available as an add-on and costs an additional $1 per two Lotto plays.
Lotto is a jackpot game that grows until someone wins!
Drawings are held on Wednesdays and Saturdays at approximately 8:59 p.m.
Next Drawing: TBA
Understanding Lotto Chances
You may already know that the chances of winning the Lotto jackpot are roughly 1 in 3.5 million, or that the overall chances of winning any prize in the Lotto game are about 1 in 20. But many people wonder how those chances of winning are determined.
Lotto is played by selecting six numbers out of 44 possible choices (the numbers from 1 to 44). During each drawing, one by one, the six numbers are randomly selected. Drawings are carefully monitored and are open to the public for viewing.
So, how does one determine what the chances of winning are for correctly matching all six numbers for any Lotto drawing? We start with the math. The formula for calculating the possibilities for matching the numbers drawn out of all the numbers in the machine is based on probability theory. So, how likely is it to get one particular outcome out of all the possible outcomes that could happen? In other words, how many different combinations of six numbers can be made out of 44 numbers?
Let's do the math; it won't be hard. The general math formula for calculating lottery chances will combine three things:
Any calculation of chances for a Lotto game involves three basic elements:
- The total number of numbers you are choosing from, which is called the field or (f);
- The number of numbers the player selects on his\her playslip, which is called the pick or (p); and
- The number of correct matches between the player's picks and the numbers drawn, which is called the match (m).
Here is the formula statisticians use to calculate the probability:
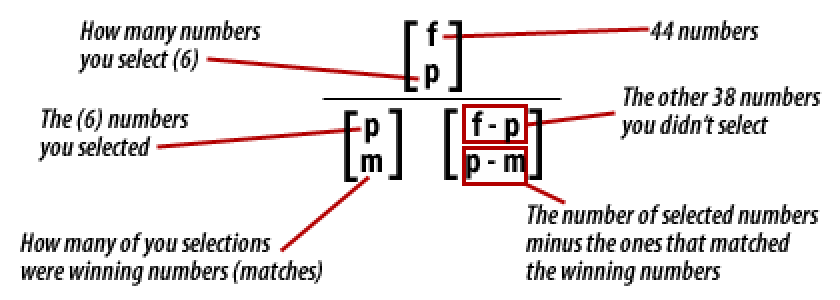
It looks complicated, but let us explain. Remember, f = the 44 numbers, p = the numbers you picked, and m = the number you correctly matched.
So, here we go. The factors or elements depicted as x over y, with ( ) around them are called binomial coefficients, and when filled in with our numbers, are what mathematicians call factorials and are depicted as x!, or a number followed by an exclamation point. What's that all about? Well, simply put, a factorial is a number multiplied progressively by all other smaller numbers until you reach 1. It looks like this:
Let's use 6 as an example; 6! would be figured as 6 x 5 x 4 x 3 x 2 x 1 = 720
In other words, we would multiply 6 times 5 times 4 times 3 times 2 times 1 to get the result. 0! is equal to 1.
So what does that have to do with Lotto? Well, 6! is all the different combinations you can theoretically make out of six numbers. Starting to make sense?
To match all six numbers and win the Lotto jackpot, here are the chances of winning:
(Remember, there are 44 numbers, and you must choose six of those numbers to play and match to win the jackpot.)
Lotto chances of winning the jackpot:

We have one six-number combination (our pick) in a possible 7,059,052 different possible combinations or outcomes. These are your chances of hitting the jackpot with any six numbers played.
But wait! Your chances are actually better than that . . .
. . . on a Lotto playslip, you must choose two sets of six numbers for each draw (two plays for $1). So, if we choose two different sets of six numbers, we actually reduce our chances by having two, not one, of the possible sets of winning combinations. So on any given playslip, if we choose different numbers on our ticket, we actually have a 1 in 3,529,526 chance of having the winning set of numbers (divide above by two).
We hope this helps to better explain the chances of winning the Lotto jackpot.
